Evaluation of preference between two generic units emerges from the joint consideration of a set of indicators or decision criteria:

(13)

In order to make choices, however, it is necessary to be able to compare pairs of units and to decide which of the two is preferred. To this end, a preference function Pk is introduced which indicates the intensity of one alternative (ai) over another (aj) for each indicator fk :

Pk: A2 --> [0, 1]. (14)

This preference function is normally considered as a function of the difference between the evaluations of ai and aj performed with the decision criterion fk :

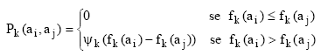(15)

where Ψ is a non-decreasing function. [4]

The PROMETHEE method yields a first synthesis of the evaluations by means of multicriteria preference indices defined as:

(16)

where πk, k=1, …, K are positive weights adding to one and reflecting the relative importance attached by a decision maker to the criteria Pk.

The indices (16) may be considered as elements in an n-order matrix S:

S = [sij] (17)

Each index sij measures the intensity of the preference for unit ai over aj considering all the criteria simultaneously.[5] To illustrate the treatment so far, Table 1 shows the evaluation of the indices (17) exemplified in the work by Brans and Vincke (1985), henceforth BV85, and frequently cited by studies on multicriteria analysis.

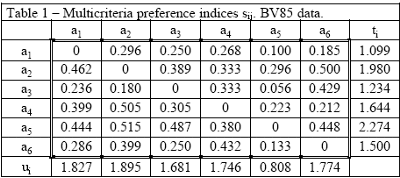
Table 1 also shows the sums:

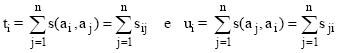(18.1)

which respectively measure the intensity of the preferences for the generic i-th unit over all the others, and the preference of all the others with respect to the i-th. It is convenient to write (18.1) in the more compact form:

t = Si e u = S’i , , (18.2)

where i denotes a vector with all the unitary components. As is evident from the exemplification in Table 1, and as normally happens in practice, the multicriteria preference indices sij satisfy neither the reversibility property (10) nor the transitive property (11), so that they are not coherent for ranking the units as in (12). Recall that the PROMETHEE I method enables to construction of a partial preference pre-order using the following rules:

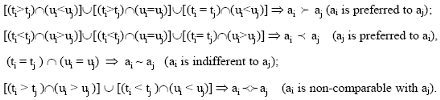
Observe that:

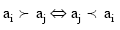(asymmetric relation);

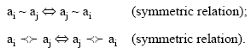
The binary relations that represent the partial pre-order generated by PROMETHEE I on the basis of the information in Table 1 and shown in Table 2 and illustrated by Figure 1.

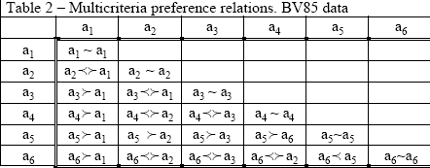
Figure 1 – Partial ranking. BV85 data

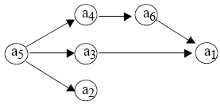
Recall finally that, according to the PROMETHEE II methods, the passage from the partial pore-order to the total one takes place by calculating the scores to assign to the individual units as differences among the overall preference intensities: h = t – u . (19)

The ranking of the units is shown in Table 3 and illustrated in Figure 2.

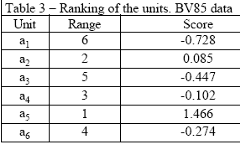
Figure 1 – Total ranking. BV85 data

---

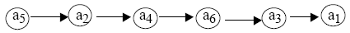
4 The analytical specifications of these preference functions are such that any problem of concrete practical relevance can be handled. Besides those initially proposed by Brans and Vincke (1985), see the preference functions devised by Diakoulaki and Koumontsos (1991) and by Huylenbroeck (1995).

5 Note that the additive nature of these indices entails as regards the choice of the functions fk, depending on whether these are associated positively or negatively with desirability, the coherence constraint stated by Merlini (2001, Theorem 1, p. 137) on the basis of the duality principle proposed by that author.

By Dr Elvio Mattioli

Next: Consideration of coherence constraints in the PROMETHEE method

Summary: Index